Abstract
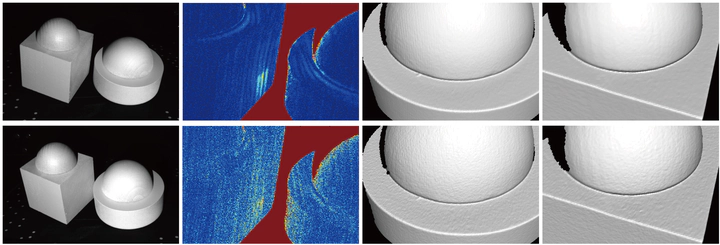
Phase shift methods have proven to be very robust and accurate for photometric 3D reconstruction. One problem of these approaches is the existence of ambiguities arising from the periodicity of the fringe patterns. While several techniques for disambiguation exist, all of them require the projection of a significant number of additional patterns. For instance, a global Gray coding sequence or several supplemental sinusoidal patterns of different periods are commonly used to complement the basic phase shift technique. In this paper we propose a new pattern strategy to reduce the total number of patterns projected by encoding multiple phases into a single sequence. This is obtained by mixing multiple equal-amplitude sinusoidal signals, which can be efficiently computed using inverse Fourier transformation. The initial phase for each fringe is then recovered independently through Fourier analysis and the unique projected coordinate is computed from the phase vectors using the disambiguation approach based on multiple periods fringes proposed by Lilienblum and Michaelis[6]. With respect to competing approaches, our method is simpler and requires fewer structured light patterns, thus reducing the measurement time, while retaining high level of accuracy.